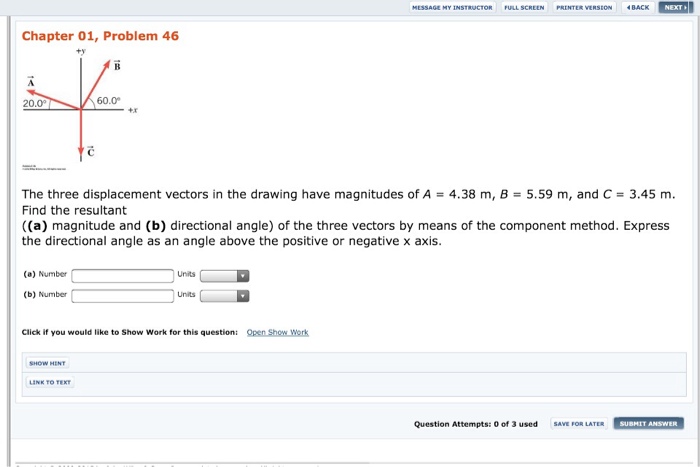
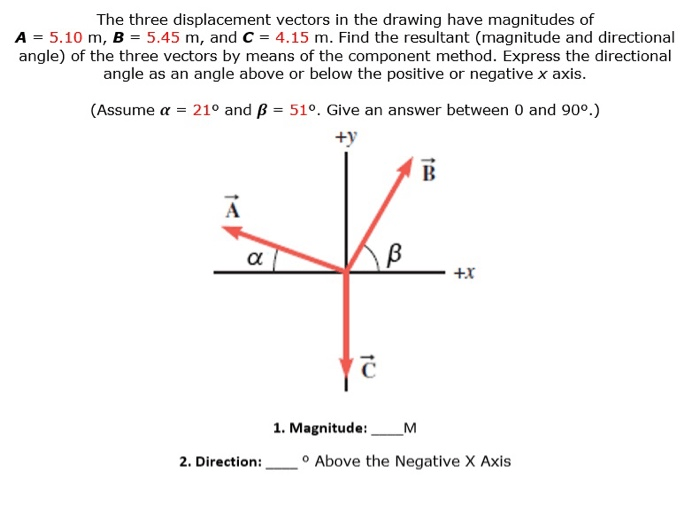
The three displacement vectors in the drawing have magnitudes of…, a fundamental concept in physics that plays a crucial role in describing the motion of objects. Displacement vectors, represented graphically as arrows, quantify the change in position of an object, providing insights into its trajectory and dynamics.
Their magnitudes, measured in units such as meters, represent the distance traveled along the displacement path.
Understanding the magnitudes of displacement vectors is essential for analyzing and solving problems in various fields, including engineering, physics, and kinematics. By comparing the magnitudes and directions of different displacement vectors, scientists and engineers can gain valuable insights into the motion of objects and systems.
Displacement Vectors
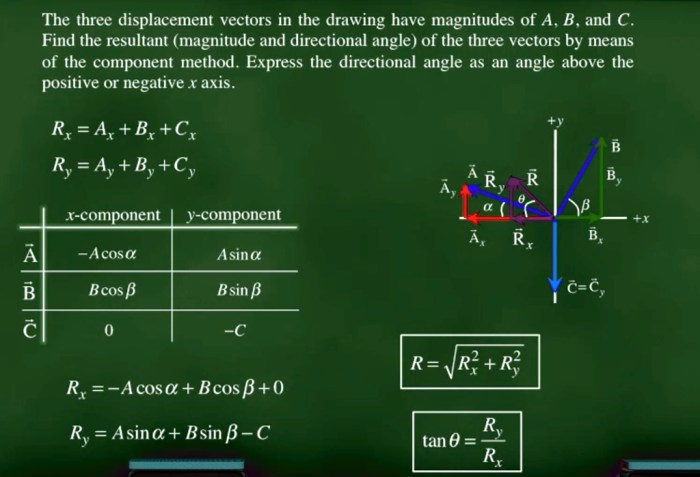
Displacement vectors are used to describe the change in position of an object. They have both magnitude and direction, and are represented graphically as arrows. The magnitude of a displacement vector is the distance between the initial and final positions of the object, and the direction is the angle between the displacement vector and the positive x-axis.
Magnitude of Displacement Vectors, The three displacement vectors in the drawing have magnitudes of
The magnitude of a displacement vector is a scalar quantity that measures the distance between the initial and final positions of an object. It is typically measured in meters (m) or kilometers (km). The magnitude of a displacement vector can be calculated using the Pythagorean theorem:
“`|d| = sqrt((x2
- x1)^2 + (y2
- y1)^2)
“`
where (x1, y1) are the coordinates of the initial position and (x2, y2) are the coordinates of the final position.
Representation of Displacement Vectors
Displacement vectors are typically represented graphically as arrows. The tail of the arrow represents the initial position of the object, and the head of the arrow represents the final position. The length of the arrow represents the magnitude of the displacement vector, and the angle between the arrow and the positive x-axis represents the direction.
Relationship between Displacement Vectors and Motion
Displacement vectors are closely related to the motion of an object. The displacement vector of an object is equal to the net change in its position over a period of time. The velocity of an object is the rate of change of its displacement vector, and the acceleration of an object is the rate of change of its velocity vector.
Applications of Displacement Vectors
Displacement vectors are used in a variety of applications, including:
- Describing the motion of objects in physics
- Calculating the distance between two points on a map
- Determining the trajectory of a projectile
Comparison of Displacement Vectors
Displacement vectors can be compared to each other to determine their relative magnitudes and directions. The magnitude of a displacement vector is a measure of the distance between the initial and final positions of an object, and the direction is a measure of the angle between the displacement vector and the positive x-axis.
Vector Addition and Subtraction
Displacement vectors can be added and subtracted using the parallelogram rule. The parallelogram rule states that the resultant vector of two vectors is the diagonal of the parallelogram formed by the two vectors. The magnitude of the resultant vector is equal to the square root of the sum of the squares of the magnitudes of the two vectors, and the direction is the angle between the resultant vector and the positive x-axis.
Question & Answer Hub: The Three Displacement Vectors In The Drawing Have Magnitudes Of
What is the significance of the magnitude of a displacement vector?
The magnitude of a displacement vector represents the distance traveled by an object along its displacement path.
How are displacement vectors used in kinematics?
Displacement vectors are used in kinematics to describe the trajectory and motion of objects, providing insights into their velocity and acceleration.
What are the applications of displacement vectors in engineering?
Displacement vectors are used in engineering to analyze the motion of objects in mechanical systems, such as robots, vehicles, and structures.